



“I started in business with an odd lot of oil and vinegar,” said a shrewd speculator. “My first customer bought $14 worth of each, paying twice as much for oil as for vinegar per gallon, and left me but one barrel. Now, see if you can guess what that barrel was worth?”
That vinegar merchant sold the 13 and 15 gal. kegs of oil at 50 cents per gal. = $14. he also sold 8, 17 and 31 gals. of vinegar at 25 cents = $14. So he had the 19 gal. barrel left, which was worth $4.75, or $9.50, according to whether it contained vinegar or oil.
2. A Rebus
The limits of my whole to scan
Is far beyond the reach of man;
Behead it and a journey take,
To prove what progress you can make;
Transpose, with rocky sides and steep
I brave the fury of the deep.
Cipher Answer. — 19, 16, 1, 3, 5.
SPACE
3. A Charade
Suppose that half a dozen of us
Were on a mountain placed;
The prospect thence, without my whole.
Would darkness seem, and waste.
Cipher Answer. — 22, 9, 19, 9, 15, 14-
VISION
5. Charade
My first— yes. I’ll straightway confess it—
’Tis a hundred to one if you guess it.
But what shall I say of my second?
Just half of a title ’tis reckoned.
My third has a personal status,
A lady, indeed, may await us.
“Good for naught,” without aid or abettors,
My whole is made up of odd letters.
Ciphers
6. The Hammock Puzzle
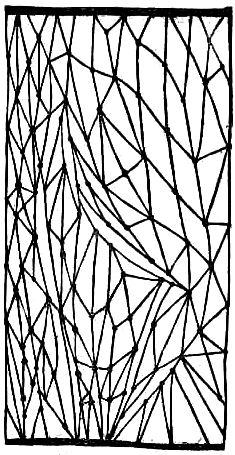
Miss Carrie Wait broke her hammock, which was suspended between two trees. On the well known axiom that a chain is no stronger than its weakest link, she says that you can readily tell her weight by finding the least number of cords you would have to cut to divide the hammock in two pieces. She says that a cord will hold exactly ten pounds. Then how much did Miss Carrie weigh?
When Miss Carrie Wait had her falling out she must have weighed 120 lbs. as the following 12 cords of the hammock broke as shown in the accompanying illustration which shows the twelve breaks, beginning at the upper left hand corner.

7.
As a companion piece to my problem of “How old was Ann?” and, by way of apology to Sister Mary, who was slighted or ignored in the public controversy of the question, we present a sketch of the reminiscent old couple who were responsible for the discussion; “You see,” remarked Grandpop “the combined ages of Mary and Ann are 44 years, and Mary is twice as old as Ann was when Mary was half as old as Ann will be when Ann is three times as old as Mary was when Mary was three times as old as Ann.” How old is Mary?
Mary’s age problem shows that she was once three times as old as Ann, so let us try 12 to 4, which shows a difference of 11 years, so, if their combined ages amount to 44, Ann is 16 yrs. 6 mos. to Mary's 27 yrs. 6 mos. Mary being twice as old as Ann was (13.9) when Mary was (24.9) half as old as Ann will be when she is (49.6) three times as old as Mary was when Mary was three times as old as Ann!
[Page 53]