


REFERENCE IS often made to the classical legend of the Delian problem, which involves the question of the duplicating or doubling the area of a cube. Philiaponus tells how the Athenians, in 432 B. C,. when suffering from the plague, consulted Plato, the pupil of Socrates, in regard to it. They conferred with the oracle at Delphi, and Apollo told them that they must double the size of the golden altar of the temple. This they were unable to do. and Plato, who was the greatest mathematician as well as philosopher, of his day, told them that they were being punished for their willful neglect of the sublime science of geometry, and deplored that they had not one man among them sufficiently wise to solve the problem.
The Delian Problem, which is neither more nor less than the duplication of the cube, is so generally confounded with that of Plato's Cubes that writers who are not up in mathematical lore get them sadly mixed. The latter is sometimes referred to as Plato’s Geometrical Numbers, and is most generally accompanied by the statement that little or nothing is known about the true conditions of the problem, and some writers maintain that its terms are lost.
It is known, however, that there was a problem referred to by ancient authors as Plato's Cubes and Geometrical Numbers, and it also is known that Plato made the science of mathematics the fundamental principle of his religious philosophy, and erected monuments in honor of the sublime truths, as he termed them.
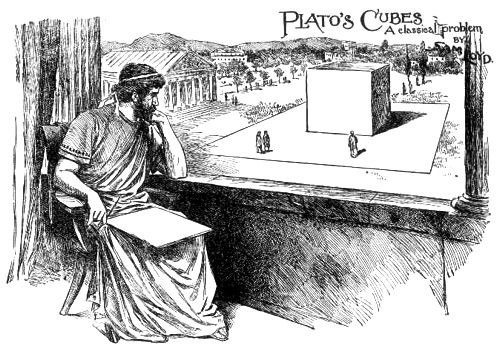
One monument, has been described as a massive cube erected in the center of a tiled plaza, and it requires no stretch of imagination to associate the monument with a problem which has been spoken of as that of the geometrical numbers. Everything is so reasonable and consistent with the requirements and history of the problem that there is no reason to doubt its ancient origin. The sketch shows Plato gazing upon a marble monument which is constructed out of a given number of smaller cubes. The monument in turn rests in the center of a square plaza, paved with cubic blocks of marble. There are just as many cubes in the pavement as there are in the monument, and they are precisely of the same size, so tell how many cubes are required to construct the monument and the square plaza upon which it stands, and you will have solved the great problem of Plato's geometrical numbers.
The majority of our mathematicians. who were to a certain extent familiar with the subject, which it is plain to be seen calls for geometrical numbers which can be squared or formed into a cube, hit upon the elementary combination of 4, viz: 4x4x4 makes a cube containing 64 cubes. This monument, therefore, might readily be placed in the centre of a square plaza of 8x8 cubes, also containing 64 cubes. Puzzlists, however, who know that the picture cuts an important figure in the puzzle, saw at a glance that the dimensions just described would not build a monument and plaza of the proportions shown in the sketch.
They, therefore, suggested a higher series of numbers, and found that 9x9x9 would form a square monument containing 729 cubes. This same number of cubes could be arranged in a 27x27 plaza which gives the correct dimensions as shown in the picture. Of course the multiples of these numbers could be employed, but 729 cubes is the only number below 1,000 which would fill the bill.
2.
How many peas would you expect to find in a pint? One (p).
What animals are as bad as Cannibals? Ant-eaters.
What was the first bet of which we have any record? The alphabet.
How would you make a Maltese Cross? Pull her tail.
What makes the ocean get angry? Because it is crossed so often.
When is a smith not a smith? When he's a-filing.
When is a mason a house? When he’s a-building.
Why is an Englishman like nineteen shillings? Because he is under a sovereign.
Why does the schoolmaster enjoy the summer? Because he keeps his days cool from nine till three.
What is the difference between a seamstress and a groom? One mends the tear and the Other tends the mare.
[Page 161]