


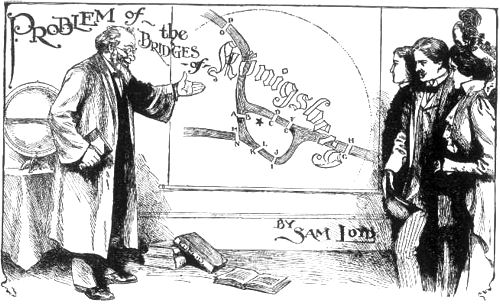
HERE IS AN ODD PUZZLE interesting not only account of the general principle involved, but because of its antiquity and the curious history connected with it. Königsberg, the second capital of Prussia, is divided by the River Pregel into four quarters, including the island of Kneiphof, as shown in the accompanying map. There are eight bridges connecting the different parts of the town, and there is a puzzle connected with them which greatly vexed the good citizens of Königsberg, over two hundred years ago.
A promenade, embracing a tour of the bridges, had always been an amusement and recreation for the young people, when according to old accounts, somehow or other the question was raised as to how long it would take to make a tour of the bridges, which led to the startling assertion that a complete tour of all bridges—without going over any bridge more than once—was impractical. It is a matter of history that a committee of young folks visited Euler, the mathematician, in 1735, and asked him to decide the point at issue. A year later Euler presented a voluminous report to the Academy of Sciences of St. Petersburg, wherein he claims to have demonstrated the impossibility of solving the problem. This decision appears in the report of the Academy, 1741, vol. 8, and has been published, in French and English by noted mathematicians, as it treats of the principle as applied to any number of bridges. Professor Ball, of Trinity College, discusses the antiquity and merits of the problem in his great work, Mathematical Recreations, but errs in ascribing its origin to Euler in 1736, and makes the remarkable statement that, “in 1759 there were, and still are, according to Baedeker but seven bridges.” The oldest records refer to eight, and our map presents an accurate tracing from Baedeker, who especially refers to the eight bridges. Euler it may be said, was a very young man in 1735, and was not the famous mathematician until nearly fifty years afterwards, so he may have fallen into the error of starting from some of the locations, which, like certain combinations of my 14-15 puzzle, would not work out,
The question of returning to the starting point, does not enter into the problem at all; it is merely a matter of proving that it is possible to start from a certain point of the town and go to another point by passing over all of the bridges but once. Then tell just how many different routes there are, and which is the shortest.
There are 416 ways of doing this trick of which the shortest route is via O-P, D-C. E-F, H-G, I-F, L-K, N-M and A-B, but as there are several million ways of not doing it, such a small matter as 416 routes may have been overlooked.
2. A CHARADE.
Of my first you have two, but here one may do,
To explain it; more need not be spoken;
In my next, deep in shade, some scores have been laid,
And when in my whole, you’r not joking!
Cipher Ans. 5, 1, 18, 14, 5, 19, 20.
EARNEST
3. A REBUS.
My first is a sign of pain,
Of sorrow or surprise;
My second it is plain
Within your kitchen lies.
My whole is found in Spain,
’Neath genial southern skies,
A fruit, —but I'll refrain,
And leave it in disguise.
Cipher Ans. 15, 18, 1, 14, 7, 5.
ORANGE
4.
Why, when you contemplate a trip into the country, should you leave the wash-hand basins behind? Because they are not ewers (yours)!
What is the greatest instance of cannibalism on record? When a rash man ate a rasher.
Why can't you make a venison pasty of buck venison? Because the pasty must be made of dough (doe).
[Page 155]