


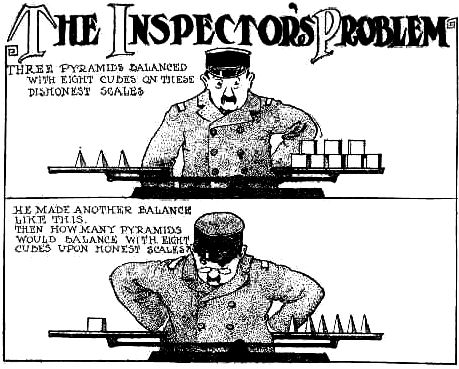
Inspector Jones' duty is to prove the correctness of weights and measures throughout the town; to see that the poor coal man is not giving half a ton too much; that the conscientious butcher is not robbing himself by giving over-weight, and that the much abused iceman is not actually defrauding the Ice Trust. But in this particular instance he is up against a ticklish problem, he finds a pair of scales which are decidedly off-center as they term it; the scales are ŌĆ£weightedŌĆØ so as to balance, although the fulcrum is not in the middleŌĆ”an error which the unsophisticated grocer is liable to overlook.
You must not judge from appearances in this case, as Benjamin Franklin wisely said, for with a puzzle-makers' license I have drawn the scales so as to give no clue to the puzzle.
In the first trial three pyramids balance with eight cubes of wood, but when he places one cube on the long arm of the lever it balances with three pyramids!
Assuming that a pyramid weighs one ounce, what should have been the true weight of the eight cubes?
Articles weighed on false scales will register out of their true weight in the same proportions as the lengths of the arms from the fulcrum point are to each other. The rule is:
ŌĆ£Weigh the articles on one side of the scales, then upon the other. Multiply the two results together and the square root of the product will be the true weight of the article.ŌĆØ
On the long arm one pyramid equaled two and two-thirds cubes, while on the short arm it weighed one-sixth of a cube.
One-sixth multiplied by two and two-thirds equals four-ninths, the square root of which is two-thirds.
Therefore, a pyramid weighs two-thirds of a square.
Assuming that a pyramid weighs one ounce, a cube would weigh one and one-half ounces, and the answer to the question, ŌĆ£What should have been the true weight of the eight cubes?ŌĆØ is twelve ounces.
2. A Charade
My firstŌĆÖs an ugly insect,
My next an ugly brute:
My whole an ugly phantom
Which naught can please nor suit.
Cipher Answer. ŌĆ”2, 21, 7, 2, 5, 1, 18.
BUGBEAR
3. Missing Words.
The blanks in this little quatrain are to be filled in with words spelled with the same seven letters:
No ________ to glory, he ________ the blows
Of the ________ that threaten his life;
Then quickly ________ to an inn that he knows,
Where the host is no ________ of strife.
The answer to the above remarkable anagram puzzle, which gives no less than five seven-letter words, to be arranged from the same letters are: Aspirer, Parries. Rapiers, Repairs and Praiser.
4. Ancient Egyptian Puzzle

Many versions have appeared in verse and prose of the story of the ancient Egyptian king who promised the hand of his beautiful daughter to the man who could shave down the sides of a perfect cube of wood to fit respectively into a square, an equilateral triangle and a circle, constructed in proportions shown in the accompanying illustration. Many scholars, scientists, mathematicians and other learned men of that time thought they could solve the problemŌĆ”but the beautiful princess died an old maid after all.
Probably our young puzzlists are cleverer than the ancient Egyptians, and may be able to find the solution. To find answers it is not necessary to actually whittle a cube of wood, simply mark out on a piece of paper three sides of the cube and indicate what cuts, if any, you would make to fit the sides into the square, the circle or equilateral triangle respectively.
5.

Tommy, Willie, Maggie and Ann bought twenty pieces of candy for twenty cents. Fudge costs four cents a box, white gum drops were four for a cent and chocolate drops two for a cent.
How did they invest their money?
The children must have bought three packages of fudge at four cents each; fifteen chocolate drops for seven and a half cents and two gum drops to make up the extra half cent.
[Page 121]