


AS A PUZZLE EDITOR I take occasion to say that now and then a correspondent will write to ask why a certain solution obtained a prize, when, according to his way of thinking, his solution was “just as good.” It may be a problem which turns upon a little mathematical point, and, whereas the winner followed the puzzler's custom of carrying the result out to only three decimals, the writer had gone to the pains of working it out to ten points, thereby producing what he considers a better answer. He employed half a dozen sheets of foolscap and worked out his solution by algebra. The winner's solution, produced by natural methods which anyone could understand, covered less than half a postal card, and clearly showed that he understood the point of the puzzle and could carry the answer out to any number of decimals, if the prize were offered for patience or endurance.
An umpire cannot always give his reasons for making an award, and so long as it is done fairly and impartially, should not open the way to discussions. It may be that the winner’s answer came several days before the others, or was clear and sharp to the point, while the others were vague and full of errors which the umpire does not desire to discuss.
These remarks are made as a hint for competitors to always give short, clear answers, as free as possible from mathematical terms. Solutions should be perfectly clear when they first go to the umpire, as no arguments or amended explanations should be accepted afterwards.
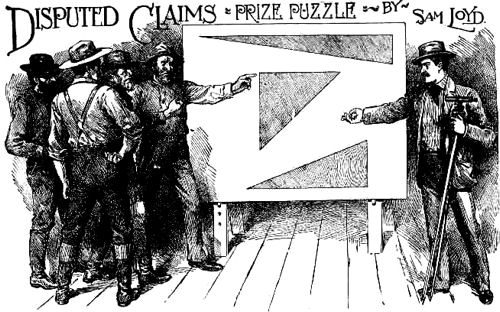
Our puzzle shows an animated dispute between some miners over their respective claims. It seems that they had obtained “patents” on some mining claims of the same size. Each claim was in the form of a right angled triangle, and all of exactly the same area, but of different dimensions, as would be the case with a triangle with a base of 35 feet, an elevation of 12 and the hypotenuse of 37, as compared with another with dimensions of 20, 87 and 29, as both contain areas of 210 feet.
The puzzle calls for a number of triangles containing 210 feet in each, with complete and definite dimensions without any two triangles being of the same shape. This puzzle gives great scope for ingenuity and perseverance, as it turns upon being able to discover the greatest number of such triangles.
How many right angled triangles of definite dimensions can you give, which will all contain the same areas, but of different dimensions on the aides?
The finding of two triangles of equal area, such as base 40, elevation 48, and the hypotenuse 148, which would contain the same area as one with an elevation of 80, a base of 84 and the hypotenuse of 116, is not difficult. To find the third right angled triangle which will also contain 3,360 square feet is so difficult that such noted mathematicians as Euler and LaPlace are said to have claimed that it was impossible to discover a fourth.
Here is the third: Elevation of 30, base 224 and hypotenuse 226.
2. A Rebus
Whether backwards or forwards I'm read,
Matters to me not a bit;
I am gentle and light, and transposed
Am ever ready and fit
3. Charade
In battle-field when front to front,
Contending armies bear the brunt,
My first is in the fray;
It e’er with quantities perplexed,
You gents may measure with my next,
Or with my total weigh.
Steel-yard.
4.
Of what part of London does a lame man remind us? Of cripple-gate (cripple gate).
Why would a tanner make a good chemist? Because he understands ox (h)ides.
Why are you better looking than a carpenter? Because he is a deal plainer (planer).
Why is the letter F like death? Because it makes all fall.
Whose chins are never shaved? Ur-chins.
Why are gloves unsaleable articles? Because they are made to be kept on the hand
[Page 113]