


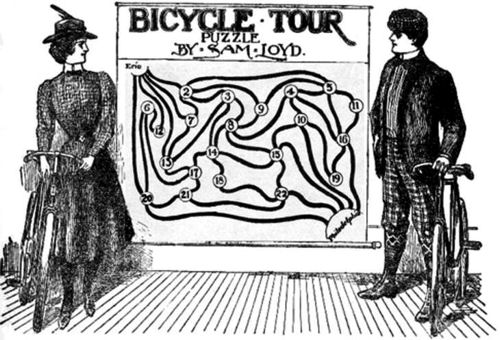
NOW THAT THE. L. A. W. and Good Roads Association have done so much toward bettering the bicycle paths of the country, it is being suggested by the press that something might be done to impart an artistic finish to many popular routes for the benefit of those who ride by the wheel or auto. Whether it is intended to round off the harsh corners and convert the straight lines into graceful curves, or to induce the malicious fiends who scatter tire-puncturing carpet tacks along the paths, to throw poppy and sunflower seeds instead, is not made clear, but the idea is a good one, and suggests the accompanying artistic map, with a pretty puzzle incidentally added.
The map shows twenty-three prominent cities of the State of Pennsylvania connected by bicycle routes of more or less artistic design. The problem is a very simple one: merely start on your summer outing and go from Philadelphia to Erie, passing through every one of the cities but once and without going over any road twice. That is all there is to it. The cities are numbered so as to enable solvers to describe their routes by a sequence of figures. In this trip the usual practice of getting there by the ŌĆ£shortest route possible, etc.,ŌĆØ will be dispensed with. Just get there without minding the cyclometer, and get an answer by giving the sequence of towns passed through.
Such of our puzzalists as completed the cross-state run, report that the only possible route by which all of the towns can be visited but once is to take them in the following sequence, according to the designated numbers: Philadelphia to 15, 22, 18, 14. 3, 8, 4, 10, 19. 16, 11, 5, 9. 2, 7, 13, 17, 21, 20, 6, 12 and then to Erie, which completes the trip after having visited the twenty-two towns.
2. A Bicycle Mishap
Here is another pretty bicycle puzzle which confronted Fred and his best girl on that same circuitous cross state tour which was to culminate at Erie. They had gone some distance when Fred's sprocket wheel broke off in such a way that, temporary repairs were out of the question, and they were so remote from any hope of other assistance that it was deemed to be a mere question of reaching home in the shortest possible time. The young lady could be depended upon to maintain a five-minute clip to the finish. Fred was an expert rider who could keep up a three-minute speed when riding upon his own wheelŌĆ”but if he rode her wheel it reduced his speed to three and a half minutes
It was evident when the accident occurred that some walking must be done. She could walk a mile in twenty minutes, handicapped by leading a wheel. He could walk a mile in fifteen minutes encumbered with the broken wheel.
An extra sprocket wheel was at home and could be attached in ten minutes, so assuming they left home at 10 A. M. and returned at precisely 6 P. M., the problem is to tell how far they have travelled by their cyclometers, if they had gone as far away from home as was possible in accordance with the conditions described.

In that story from actual experience it was made clear, by giving the facts Just as they occurred, so as to appeal to the common sense as well as the mathematical powers of the solvers. It was told that Fred and his best girl started out on a century run one bright morning at 10 o'clock. They went at a five-minute clip and had gone some distance when Fred broke his sprocket wheel in a way which permitted of no temporary repairs. They were compelled to return. He could maintain a three-minute gait on his own wheel, but on her wheel could not do better than three and a half minutes. She could walk, leading the broken wheel, at a speed of a mile in twenty minutes, and he could do the same in fifteen minutes. Everything was favorable, and it was also stated that a new sprocket wheel was ready at home, and could be replaced in ten minutes. Now then, if they left home at 10 A. M., and assuming that they did the best that circumstances permitted, according to the conditions mentioned, and both got home at exactly 6 o'clock, what would their respective cyclometers register if they had gone as far as possible up to the point of the accident?
The accident occurred when he had gone 33 3/17 miles at the speed of five minutes [i.e. five minutes per mile, jws] ŌĆ” 2 hr. 45 15/17 min.; at 33 3/17 miles he returns, at 3 1/2 minute speed ŌĆ” 1 hr. 56 2/17 min.; 23 5/17 miles back to meet the lady, 3 1/2-minute speed ŌĆ” 1 hr. 21 9/17 min.; mending wheel, 10 min.; 5 miles on his own wheel, at 3-minute gait ŌĆ” 15 min.; 18 15/17 min., 9 15/17 miles walking, 20-minute speed ŌĆ” 3 hr. 17 11/17 min.; 23 5-17 miles riding home, five minute speed ŌĆ” 1 hr. 56 8/17 min.; total, 8 hours.
Her record ŌĆ” 33 3-17 miles to accident, five-minute speed ŌĆ” 2 hr. 45 15/17 miles walking, 20-minute speed ŌĆ” 3 hr. 17 11/17 min.; 23 5/17 miles riding home, five-minute speed ŌĆ” 1 hr. 56 8/17 min.; total 8 hours.
Many overlooked the point that she might ride on while he was fixing his new sprocket wheel, and that the time or distance is not affected by the loss of the ten minutes. Her cyclometer should register 112 16-17 miles, and his but 66 6-17, leaving the matter as to which has done the hardest work and is the most tired an open question.
[Page 11]