


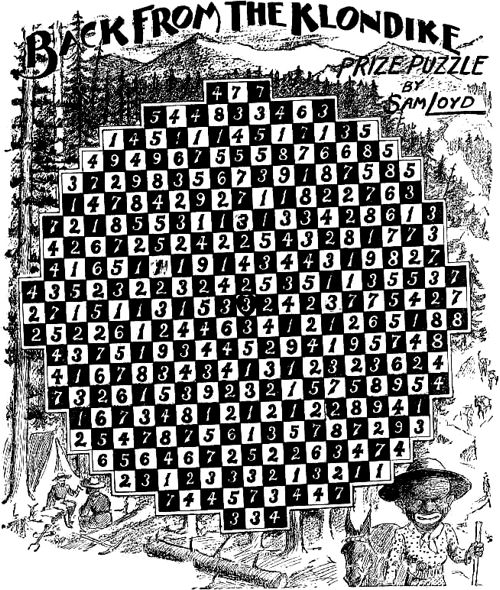
Euler, the great mathematician, discovered a rule for solving all manner of maze puzzles, which, as all good puzzlists know, depends chiefly upon working backwards. This puzzle. however, was built purposely to defeat Euler's rule and out of many attempts is probably the only one which thwarts his method.
Start from that heart in the center, and go three steps in a straight line in any one of the eight directions, north, south, east or west, or on the bins, as the ladies cay, northeast, northwest, southeast or southwest. When you have gone three steps in a straight line, you will reach a square with a number on it, which indicates the second day's journey, as many steps as it tells, in a straight line in any one of the eight directions. From this new point when reached, march on again according to the number indicated, and continue on, following the requirements of the numbers reached, until you come upon a square with a number which will carry you just one step beyond the border, when you are supposed to be out of the woods and can holler all you want, as you will have solved the puzzle.
That puzzling return trip from the Klondike proved to be no easy task for our young puzzlists, and but few succeed in getting out of the woods with their treasure. For the benefit of such as could not escape the endless whirlpool of numbers which held them in its vortex we will say that the only escape leads through the backward and forward sequence of S. W. to 4, S. W. 6, M. E. 6, M. E. 2, M. E. 5, S. W. 4, S. W. 4, S. W. 4, and a bold strike via N. W. to liberty!
Those who failed to master it readily discovered that one false step at any stage of the game throws one into the whirlpool from which there is no egress.
[Page 106]